hypothesis testing wald statistic cobb douglas|wald test statistics : factory First, the Cobb-Douglas captures to a first approximation (at least.) some nonlinearities and substi tution characteristics among production inputs hypothesized WEBLa Familia sacana es una de las familias mas populares en el mundo de los comics porno, por su suculenta manera de ver la vida, todo es follar y sentir placer :v.
{plog:ftitle_list}
Resultado da Novinhas Lesbicas - sexovideo.tv 3 min. 3 min Flagrasporno - 360p. Lesbicas Novinhas de 20 anos se chupando 8 min. 8 min Paulo Freitas55 - 1080p. Novinhas safadas lésbicas que dão para o amigo - Nanah Haze - Marianna Angel - Frotinha Porn Star 11 min. 11 min Ws Producoes - 155.5k .
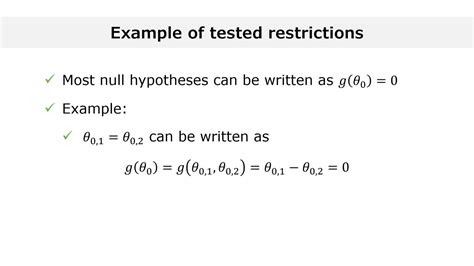
The Wald Test Statistic. Wn = n(Cbθn − h)0(C I(θ) d. −1. C0)−1(Cbθn − h) Again, null hypothesis is H0 : Cθ = h. Matrix C is r × k, r ≤ k, rank r. All we need is a consistent estimator of I(θ) I(bθ) .
The previous version of the test is often called the Wald test, and it measures the discrepancy between r, the value(s) asserted by the null, and R ^, the estimates obtained from the model.First, the Cobb-Douglas captures to a first approximation (at least.) some nonlinearities and substi tution characteristics among production inputs hypothesized Linear hypothesis test on the parameters can be done with t_test or f_test or wald_test methods of the results instance. –the test statistic is another random variable that is a function of the data and null hypothesis. T denotes the random variable test statistic; t denotes the single realization of the test statistic; .
In statistics, the Wald test (named after Abraham Wald) assesses constraints on statistical parameters based on the weighted distance between the unrestricted estimate and its .Wald (and score) tests • MLEs have an approximate multivariate normal sampling distribution for large samples (Thanks Mr. Wald.) • Approximate mean vector = vector of true parameter .
wald test statistics
The Wald statistic and Wald confidence interval are based on the asymptotic distribution of the MLE, as given in Theorem 1 and Note 3. The statistic can be used to test whether our true .Alternate Interpretation of Test Statistic When conducting a hypothesis test, we consider null hypotheses of the form: H 0: R = r The previous version of the test is often called the Wald test, and it measures the discrepancy between r, the value(s) asserted by the null, and R ^, the estimates obtained from the model.In statistics, the Wald test (named after Abraham Wald) assesses constraints on statistical parameters based on the weighted distance between the unrestricted estimate and its hypothesized value under the null hypothesis, where the weight is the precision of the estimate. [1] [2] Intuitively, the larger this weighted distance, the less likely it is that the constraint is true.
The Wald and likelihood ratio (LR) tests are shown to have incorrect size. A one-sided LR test and a test of the significance of the third moment of the OLS residuals are suggested as alternatives, and are shown to have correct size, with the one-sided LR test having the better power of the two.At the 5% level of significance we should reject if the realisation of our test statistic exceeds c.05 = 5.99 (test asymptotic χ 2 2 under H 0 . Since our reali- sation is smaller, we will not reject the null that the elasticity of labour and capital are respectively 0.7 and 0.3. It is noted that the sample size is quite small (asymptotic validity of the test statistic).The null hypothesis. We assume that an unknown -dimensional parameter vector has been estimated by ML. We want to test the null hypothesis that equations (possibly nonlinear) are satisfied: where is a vector valued function, with .. The lecture on hypothesis testing in maximum likelihood framework explains that the most common null hypotheses can be written in this form.(Wt) is zero versus the alternative hypothesis that it is not equal to zero. (c) At the 1 % level of significance, test for the overall significance of the above estimated regression. 3. In an application of the Cobb-Douglas production function the following results were obtained: 1n Yi = 2.3542 + 0.9576(1nX2i) + 0.8242(1nX3i) (0.3022) (0.3571)
A new Wald-type statistic is proposed for hypothesis testing based on Bayesian posterior distributions under the correct model specification. The new statistic can be explained as a posterior version of the Wald statistic and has several nice properties. First, it is well-defined under improper prior distributions.
The Wald test statistic is a method used to assess the significance of individual coefficients in statistical models, especially within the context of maximum likelihood estimation. It compares the estimated parameter to its standard error, essentially checking whether the parameter is significantly different from zero or some null value. This test is closely related to asymptotic . The second method is based on statistical decision theory. The idea begins with Bernardo and Rueda (2002, BR hereafter) where they demonstrate that the BF can be regarded as a decision problem with a simple zero–one loss function when it is used for point hypothesis testing. It is this zero–one loss that leads to Jeffreys–Lindley–Bartlett’s paradox. Preliminary Testing of the Cobb-Douglas Production Function and Related Inferential Issues March 2017 Communication in Statistics- Simulation and Computation 46(1):469-488 This suggests that there is strong evidence of unity labour elasticity of output at the 5% significance (2 Marks) Wald Test: Equation: Untitled Test Statistic Value df Probability t-statistic 3.339495 38 0.0019 F-statistic 11.15223 (1, 38) 0.0019 Chi-square 11.15223 1 0.0008 Null Hypothesis: C(2)=1
Wald test. Let be the estimate of a parameter , obtained by maximizing the log-likelihood over the whole parameter space : The Wald test is based on the following test statistic: where is the sample size and is a consistent estimate of the asymptotic covariance matrix of (see the lecture entitled Maximum likelihood - Covariance matrix estimation).558 model and assess them in one step, and takes into account statistical noise, s o the differences among firms are given not only due to inefficiency, but also because of other stochastic factors .
One of the first to estimate cost models in the electric industry was Marc Nerlove (1963), who employed the dual to the Cobb-Douglas production function, which was introduced in the seminal paper by Charles Cobb and Paul Douglas in 1928.In general, a production function summarizes the relationship among inputs and output.A Cobb-Douglas production function analysis . . The Wald test result explains whether we should reject or accepts the null . In order to test the hypothesis or literature explained. We .The Wald Test Statistic W n = n(Cbθ n − h)0(CId(θ) −1 n C 0)−1(Cθb n − h) I Again, null hypothesis is H 0: Cθ = h I Matrix C is r ×k, r ≤ k, rank r I All we need is a consistent estimator of I(θ) I I(bθ) would do I But it’s inconvenient I Need to .Wald Test for a Linear Hypothesis Description. Computes a Wald Test for a parameter \bold{\theta} with respect to a linear hypothesis \bold{R} \bold{\theta}=\bold{c}. . A vector containing the \chi^2 statistic (X2), degrees of freedom (df), p .
Wald Test Statistic. The Wald Test Statistic for testing H 0: Rβ= qvs. H . Simple F-test in a Cobb-Douglas model . With a p-value of 0.23 we fail to reject the null hypothesis. However, the F-test statistic only follows the F-distribution if ϵis Normally distributed. Test the Normality assumption using the Jarque-Bera
Compare the Wald statistic with a critical value: The wald.test() function returns the Wald statistic and the p-value of the test. The p-value is the probability of obtaining a test statistic as extreme as, or more extreme than, the observed statistic under the null hypothesis.•Hypothesis Testing Under Normality •Maximum Likelihood Estimator . statistic defined as is distributed as F(J,n-K), the F distribution with J and n-K degrees of freedom. 11 2 . • Cobb-Douglas Cost Function for Electricity Generation (Christensen and Greene [1976])
The Wald test The Wald test uses test statistic: T(Y) = ^ 0 SEc: The recipe: I If the true parameter was 0, then the sampling distribution of the Wald test statistic should be approximately N(0;1). I Look at the observed value of the test statistic; call it T obs. I Under the null, jT obsj 1:96 with probability 0.95. I So if we reject the null when jT obsj>1:96, the size of .The paper "Cobb-Douglas Function of Production " is a perfect example of a micro and macroeconomic report. The economy can be thought of as a financial system of a . In Table 5, there is Wald Test results on the hypothesis. The coefficients of capital (C2) and labor (C3), when summed tend to 1 but not 0. . (F-statistic) 0.000000 Appendix 6 .
WALD test done during the final stage identifies the short-run causality among all vari- ables (see Table 6 for results). The null hypothesis is rejected if the probability value is less than the .
Explain in an outline how to test the null hypothesis. (Hint: Follow the steps in hypothesis. testing in our discussion in statistical inference.) d. . The Cobb-Douglas. View the full answer. Step 2. Unlock. Step 3. Unlock. Step 4. Unlock. Answer. Unlock. Previous question Next .
Section 2 reviews existing posterior-based statistics for hypothesis testing in the statistical decision framework. Section 3 develops the new statistic and establishes its large sample theory under the correct model speci cation. Section 4 develops a version of the test statistic that is robust under model misspeci cation We are interested in testing the joint hypothesis H 0: β 1 = 0.3, β 2 = 0.7 against the alternative H 1 : β 1 = 0.3, β 2 = 0.7. The Wald test we can use to test this hypothesis is given by d ′ (d Var (d | X)) − 1 d a ∼ χ 2 J under H 0 where d = R ˆ β − c is the discrepancy vector. (a) Write the null hypothesis in terms of R β .State and check the assumptions for a hypothesis test. Each hypothesis test has its own assumptions. They will be stated when the different hypothesis tests are discussed. Find the sample statistic, test statistic, and p-value. This depends on what parameter you are working with, how many samples, and the assumptions of the test.
wald test examples pdf
DeviantART: Jay-Marvel - Where I’ll be posting anything that abides by their rules. Hentai-Foundry: Jay-Marvel - I’ll try to post often on here but the submission system can tend .
hypothesis testing wald statistic cobb douglas|wald test statistics